The Associative Property of Addition.

The Associative Property of Addition: When three or more numbers are added, the sum is the same, regardless of the order of addition.
For example (2 + 3) + 1 = 2 + (3 + 1).
Pretty simple.
In other words: it doesn't matter how you add them, the totals are always same.
(O O + O O O) + O is the same as O O + (O O O + O)
because
OOOOOO = OOOOOO
and six equals six, (6 = 6) everyday of the week and twice on Sundays.
This is so obvious with blocks your younglings may look at you funny when you explain it to them, math concepts are visually obvious when presented with manipulatives.
Now just for fun (and this has very little to do with The Associative Property of Addition unless you set it up correctly) here is a problem that most little pupils will find VERY difficult unless they have the blocks and some guidance from you.
Bill, Ted and Mary have some sugary sweets that will make you hyper active, rot your teeth out and cause adult onset diabetes if you eat too many of them.
Together Bill and Ted have Five.
All together Bill, Ted and Mary have six.
Together Ted and Mary have four.
All together Bill, Ted and Mary still have six.
How many sickly, sugary sweets that will make you hyper active, rot your teeth out and cause adult onset diabetes if you eat too many of them do each of them have?
B + T = 5
(B + T) + M = 6
T + M = 4
B + (T + M) = 6
(B + T) + M = B + (T + M)
Get the blocks out and this problem is easy to solve. Without them it can be a little confusing...
Even with this terrible picture it becomes OBVIOUS:
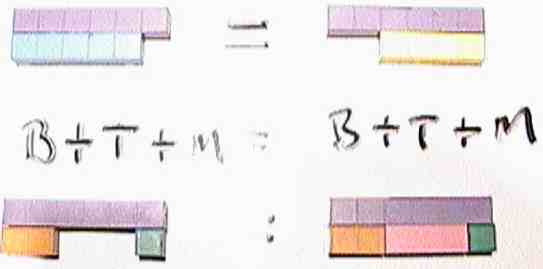
This is my impersonation of a math text book, because The Associative Property of Addition is a super easy concept but the above problem disguises it as something hard.
K.I.S.S.
Just put the blocks on a white board or piece of paper and group them with brackets...

(2 + 3) + 1 = 2 + (3 + 1)
Any youngling understands this. This concept has a name, we call it The Associative Property of Addition. It means this is the same as this...(point to the blocks on one side and then the other). You may also spend a few moments on the equals sign itself. The equals sign means SAME. They may not look same but they are...we have lots of ways to see through any disguises the numbers might put on. The equals sign is your best friend because the equals sign NEVER lies...
Next time tell that story using apples...or maybe oranges. On the password protected problem solving page there are links pages that show you how to use Ten Apples Up On Top to teach multivariable problem solving. As you can see from Bill, Ted and Mary's story problem base ten blocks make them visually obvious.
I must study politics and war that my sons may have liberty to study mathematics and philosophy. ~John Adams
This quote almost makes me cry. We have squandered our birthright and squandered the gifts our founding fathers gave us that were paid for with blood...but i digress.
"An excessive study of mathematics absolutely incapacitates the mind..." ~Sir William Hamilton
Only if the mind is tiny.
"If I had inherited a fortune I should probably not have cast my lot with mathematics." ~Joseph-Louis Lagrange
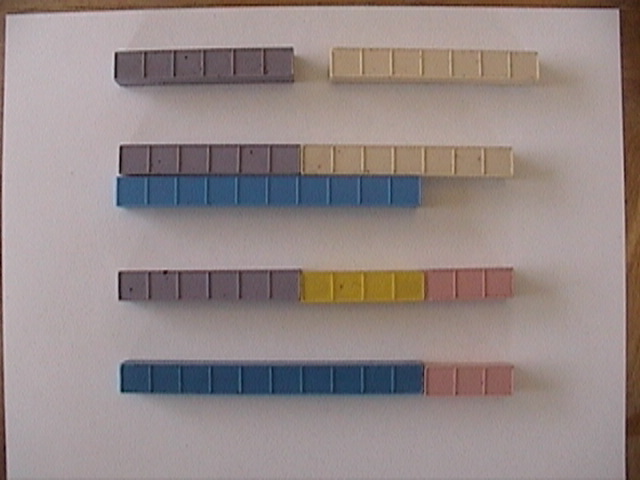 The associative property of addition is taken for granted using base 10 blocks. Of course 6 + 4 + 3 = 3 + 4 + 6, but we were adding 6 + 7. Here six takes four from seven. But seven could just as easily take three from six. Either way it's 13.
The associative property of addition is taken for granted using base 10 blocks. Of course 6 + 4 + 3 = 3 + 4 + 6, but we were adding 6 + 7. Here six takes four from seven. But seven could just as easily take three from six. Either way it's 13.The Four Properties of Addition
There are four properties of addition: they are the commutative, associative, additive identity and distributive properties.
Commutative property: When two numbers are added, the sum is the same regardless of the order of the addends.
For example 3 + 2 = 2 + 3
Algebraic: x + y = y + x
Associative Property: When three or more numbers are added, the sum is the same regardless of the order of addition.
The property states that for all real numbers a, b, and c, their sum is always the same, regardless of their grouping:
(a + b) + c = a + (b + c)
For example (1 + 2) + 3 = 1 + (2 + 3)
Algebraic: (x + y) + z = x + (y + z)
Additive Identity Property: The sum of any number and zero is the original number.
For example 1 + 0 = 1.
Algebraic: x + 0 = x (Crucially important concept especially when you understand 0 = x - x.) Hero zero! Helps solve equalities.
Distributive property: The sum of two numbers times a third number is equal to the sum of each addend times the third number.
For example 4(6 + 3) = (4)(6) + (4)(3)
Algebraic: x(y + z) = xy + xz
(Another crucially important concept that is deceptively simple. Also note if you have any sense at all you'd just multiply (4)(9) and get 36, add 6 + 3, It's easier, instead of adding 24 and 12. But that's Order Of Operations and I'm trying to show you the distributive property...
Crewton Ramone has a FaceBook page too. Lots of fun memes FB stuff there. There is also a secret page. But it isn't free.

Get this book if you have children 7 and under...look inside here. You can get it and all of my books without a password for just $19.99.
"Great book for teaching how to use the blocks! Colorful, clear pictures and cute rhymes make the book fun to read and play around with. We printed the book out, and my 5yo loves how many of the block pictures are big enough to put his blocks directly on top of the pictures. The text plays fast and loose with niceties like punctuation, but is engaging when read aloud.
Most of the book focuses on playing with addition facts up to 10, which gives a solid foundation. But it also delves briefly into such topics as square roots, place value, addition of multi-digit numbers, and a glimpse at multiplication. And in true Crewton Ramone fashion, problem solving with 'x' (basic algebra) is sprinkled throughout. A great intro to playing with math." ~CS, GA.

